Can you solve Martin Gardner’s best mathematical puzzles?

The maestro of recreational maths was born 100 years ago today. Here we celebrate his birthday with eight of his most celebrated puzzles

Martin Gardner

Here’s something puzzling.

A few years before he died, aged 95 in 2010, I interviewed Martin Gardner at his home in Norman, Oklahoma.

Gardner was a journalist, a novelist, a magician, a philosopher and one of the earliest public debunkers of pseudoscience.

Yet he was probably best known – and most loved – for popularising mathematical puzzles.

In his monthly column Mathematical Games, which he wrote in Scientific American between the 1950s and the 1980s, he introduced many brainteasers as well as giving old classics new twists.

I asked him if he enjoyed solving puzzles?

“Not particularly,” he replied. “I’m not very good at it, really.”

His answer threw me. It was as if Pelé had told me he didn’t like playing football, or Jamie Oliver that he wasn’t bothered about food.

Yet when we continued the discussion I realised that my analogies were wrong. There is a difference between being good at puzzles and appreciating a good puzzle.

“A puzzle in a sense models what all scientists are doing,” he said. “They are trying to solve puzzles about the nature of the universe.”

Puzzles both provoke creative thinking and are a starting point for interesting research.

“Puzzles can lead you into almost every branch of mathematics,” he added.

And Martin Gardner was without parallel in being able to show how true that was.

Gardner wrote dozens of books on puzzles and recreational maths – here are eight puzzles taken from them. I’ll post the answers in a few days … but by then you will have solved them all, right?

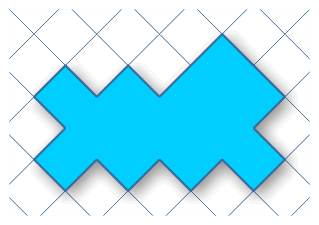
1. Crazy cut

You are to make one cut (or draw one line) – of course it needn’t be straight – that will divide the figure into two identical parts.

2. The coloured socks

Ten red socks and ten blue socks are all mixed up in a dresser drawer. The 20 socks are exactly alike except for their colour. The room is in pitch darkness and you want two matching socks. What is the smallest number of socks you must take out of the drawer in order to be certain that you have a pair that match?

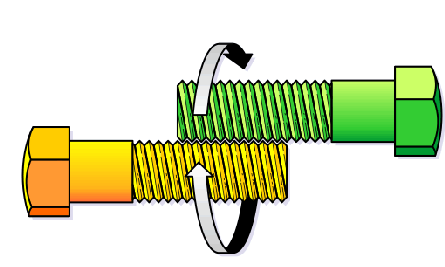
3. Twiddled bolts

Two identical bolts are placed together so that their helical grooves intermesh as shown below. If you move the bolts around each other as you would twiddle your thumbs, holding each bolt firmly by the head so that it does not rotate and twiddling them in the direction shown, will the heads

(a) move inward,

(b) move outward, or

(c) remain the same distance from each other?

4. The fork in the road

A logician vacationing in the South Seas finds himself on an island inhabited by two proverbial tribes of liars and truth-tellers. Members of one tribe always tell the truth, members of the other always lie. He comes to a fork in a road and has to ask a native bystander which branch he should take to reach a village. He has no way of telling whether the native is a truth-teller or a liar. The logician thinks a moment, then asks one question only. From the reply he knows which road to take. What question does he ask?

5. Three squares

Using only elementary geometry (not even trigonometry), prove that angle C equals the sum of angles A and B.

6. Cutting the pie

With one straight cut you can slice a pie into two pieces. A second cut that crosses the first one will produce four pieces, and a third cut can produce as many as seven pieces. What is the largest number of pieces that you can get with six straight cuts?

7. The mutilated chessboard

The props for this problem are a chessboard and 32 dominoes. Each domino is of such size that it exactly covers two adjacent squares on the board. The 32 dominoes therefore can cover all 64 of the chessboard squares. But now suppose we cut off two squares at diagonally opposite corners of the board and discard one of the dominoes.

Is it possible to place the 31 dominoes on the board so that all the remaining 62 squares are covered? If so, show how it can be done. If not, prove it impossible.

Domino dancing. Courtesy ThinkFun

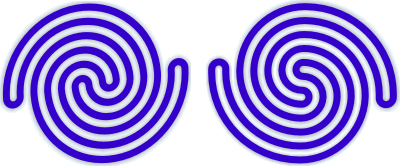
8. The two spirals

One of these spirals is formed with a single piece of rope that has its ends joined. The other spiral is formed with two separate pieces of rope, each with joined ends.

Can you tell which is which by using only your eyes? No fair tracing the lines with a pencil.

Sourse: theguardian