CISSOID OF DIOCLES

 

Curve studied by Diocles, 180 BC; Fermat; Huygens. From the Greek Kissos: ivy, probably in reference to the nervures...  Diocles (2nd century BC): Greek mathematician.

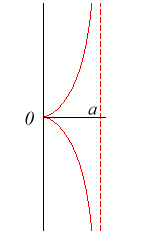Polar equation:  . Cartesian equation:   (the curve  is studied here).  Rational circular cubic with a cuspidal point. Rational Cartesian parametrization: , i.e.  (where t = tanq). Cartesian tangential angle:  . Curvilinear abscissa: , . Radius of curvature: . Area between the curve and its asymptote:  .

Read more »