Suppose I roll a die and ask you to guess the number I roll. What's the chance of you getting it right?
As long as the die is fair, so all numbers are equally likely to be rolled, the probability of you guessing correctly is 1/6. But what if I tell you, before I uncover the result, that the number I have rolled is odd? Then there are only three possible outcomes: 1, 3, and 5. The chance of you guessing correctly is now 1/3. Halving the number of possible outcomes has doubled your chance of getting it right.
What we have been looking at here is a conditional probability: the probability of you guessing correctly given that I told you that I have rolled an odd number.
More generally, given to events 
and 
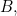the conditional probability of 
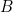occurring given that has occurred is written as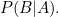 
To work it out, you can use the formula
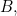and the conditional probability of 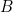occurring given that has occurred is written as 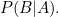To work it out, you can use the formula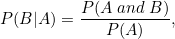
where 
is the probability of both 
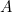and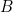 
occurring.
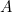is the probability of both and 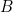occurring.
Δεν υπάρχουν σχόλια:
Δημοσίευση σχολίου